Every statistical software procedure that dummy codes predictor variables uses a default for choosing the reference category.
This default is usually the category that comes first or last alphabetically.
That may or may not be the best category to use, but fortunately you’re not stuck with the defaults.
So if you do choose, which one should you choose? (more…)
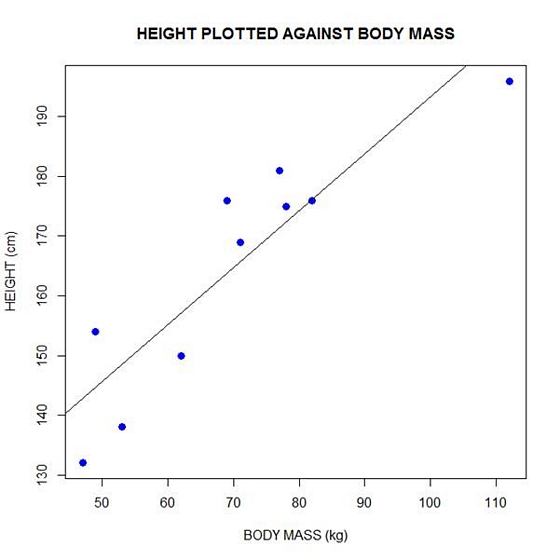
In Part 2 of this series, we created two variables and used the lm() command to perform a least squares regression on them, treating one of them as the dependent variable and the other as the independent variable. Here they are again.
height = c(176, 154, 138, 196, 132, 176, 181, 169, 150, 175)
bodymass = c(82, 49, 53, 112, 47, 69, 77, 71, 62, 78)
Today we learn how to obtain useful diagnostic information about a regression model and then how to draw residuals on a plot. As before, we perform the regression.
lm(height ~ bodymass)
Call:
lm(formula = height ~ bodymass)
Coefficients:
(Intercept) bodymass
98.0054 0.9528
Now let’s find out more about the regression. First, let’s store the regression model as an object called mod and then use the summary() command to learn about the regression.
mod <- lm(height ~ bodymass)
summary(mod)
Here is what R gives you.
Call:
lm(formula = height ~ bodymass)
Residuals:
Min 1Q Median 3Q Max
-10.786 -8.307 1.272 7.818 12.253
Coefficients:
Estimate Std. Error t value Pr(>|t|)
(Intercept) 98.0054 11.7053 8.373 3.14e-05 ***
bodymass 0.9528 0.1618 5.889 0.000366 ***
Signif. codes: 0 ‘***’ 0.001 ‘**’ 0.01 ‘*’ 0.05 ‘.’ 0.1 ‘ ’ 1
Residual standard error: 9.358 on 8 degrees of freedom
Multiple R-squared: 0.8126, Adjusted R-squared: 0.7891
F-statistic: 34.68 on 1 and 8 DF, p-value: 0.0003662
R has given you a great deal of diagnostic information about the regression. The most useful of this information are the coefficients themselves, the Adjusted R-squared, the F-statistic and the p-value for the model.
Now let’s use R’s predict() command to create a vector of fitted values.
regmodel <- predict(lm(height ~ bodymass))
regmodel
Here are the fitted values:
1 2 3 4 5 6 7 8 9 10
176.1334 144.6916 148.5027 204.7167 142.7861 163.7472 171.3695 165.6528 157.0778 172.3222
Now let’s plot the data and regression line again.
plot(bodymass, height, pch = 16, cex = 1.3, col = "blue", main = "HEIGHT PLOTTED AGAINST BODY MASS", xlab = "BODY MASS (kg)", ylab = "HEIGHT (cm)")
abline(lm(height ~ bodymass))
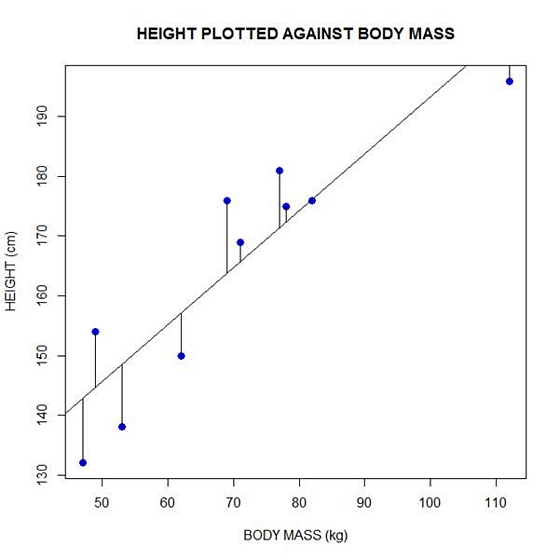
We can plot the residuals using R’s for loop and a subscript k that runs from 1 to the number of data points. We know that there are 10 data points, but if we do not know the number of data we can find it using the length() command on either the height or body mass variable.
npoints <- length(height)
npoints
[1] 10
Now let’s implement the loop and draw the residuals (the differences between the observed data and the corresponding fitted values) using the lines() command. Note the syntax we use to draw in the residuals.
for (k in 1: npoints) lines(c(bodymass[k], bodymass[k]), c(height[k], regmodel[k]))
Here is our plot, including the residuals.
In part 4 we will look at more advanced aspects of regression models and see what R has to offer.
About the Author: David Lillis has taught R to many researchers and statisticians. His company, Sigma Statistics and Research Limited, provides both on-line instruction and face-to-face workshops on R, and coding services in R. David holds a doctorate in applied statistics.
See our full R Tutorial Series and other blog posts regarding R programming.
Just recently, a client got some feedback from a committee member that the Analysis of Covariance (ANCOVA) model she ran did not meet all the assumptions.
Specifically, the assumption in question is that the covariate has to be uncorrelated with the independent variable.
This committee member is, in the strictest sense of how analysis of covariance is used, correct.
And yet, they over-applied that assumption to an inappropriate situation.
Analysis of Covariance was developed for experimental situations and some of the assumptions and definitions of ANCOVA apply only to those experimental situations.
The key situation is the independent variables are categorical and manipulated, not observed.
The covariate–continuous and observed–is considered a nuisance variable. There are no research questions about how this covariate itself affects or relates to the dependent variable.
The only hypothesis tests of interest are about the independent variables, controlling for the effects of the nuisance covariate.
A typical example is a study to compare the math scores of students who were enrolled in three different learning programs at the end of the school year.
The key independent variable here is the learning program. Students need to be randomly assigned to one of the three programs.
The only research question is about whether the math scores differed on average among the three programs. It is useful to control for a covariate like IQ scores, but we are not really interested in the relationship between IQ and math scores.
So in this example, in order to conclude that the learning program affected math scores, it is indeed important that IQ scores, the covariate, is unrelated to which learning program the students were assigned to.
You could not make that causal interpretation if it turns out that the IQ scores were generally higher in one learning program than the others.
So this assumption of ANCOVA is very important in this specific type of study in which we are trying to make a specific type of inference.
But that’s really just one application of a linear model with one categorical and one continuous predictor. The research question of interest doesn’t have to be about the causal effect of the categorical predictor, and the covariate doesn’t have to be a nuisance variable.
A regression model with one continuous and one dummy-coded variable is the same model (actually, you’d need two dummy variables to cover the three categories, but that’s another story).
The focus of that model may differ–perhaps the main research question is about the continuous predictor.
But it’s the same mathematical model.
The software will run it the same way. YOU may focus on different parts of the output or select different options, but it’s the same model.
And that’s where the model names can get in the way of understanding the relationships among your variables. The model itself doesn’t care if the categorical variable was manipulated. It doesn’t care if the categorical independent variable and the continuous covariate are mildly correlated.
If those ANCOVA assumptions aren’t met, it does not change the analysis at all. It only affects how parameter estimates are interpreted and the kinds of conclusions you can draw.
In fact, those assumptions really aren’t about the model. They’re about the design. It’s the design that affects the conclusions. It doesn’t matter if a covariate is a nuisance variable or an interesting phenomenon to the model. That’s a design issue.
So what do you do instead of labeling models? Just call them a General Linear Model. It’s hard to think of regression and ANOVA as the same model because the equations look so different. But it turns out they aren’t.
If you look at the two models, first you may notice some similarities.
But wait a minute, Karen, are you nuts?–there are no Xs in the ANOVA model!
Actually, there are. They’re just implicit.
Since the Xs are categorical, they have only a few values, to indicate which category a case is in. Those j and k subscripts? They’re really just indicating the values of X.
(And for the record, I think a couple Xs are a lot easier to keep track of than all those subscripts. Ever have to calculate an ANOVA model by hand? Just sayin’.)
So instead of trying to come up with the right label for a model, focus instead on understanding (and describing in your paper) the measurement scales of your variables, if and how much they’re related, and how that affects the conclusions.
In my client’s situation, it was not a problem that the continuous and the categorical variables were mildly correlated. The data were not experimental and she was not trying to draw causal conclusions about only the categorical predictor.
So she had to call this ANCOVA model a multiple regression.
A Linear Regression Model with an interaction between two predictors (X1 and X2) has the form:
Y = B0 + B1X1 + B2X2 + B3X1*X2.
It doesn’t really matter if X1 and X2 are categorical or continuous, but let’s assume they are continuous for simplicity.
One important concept is that B1 and B2 are not main effects, the way they would be if (more…)
One of the most common causes of multicollinearity is when predictor variables are multiplied to create an interaction term or a quadratic or higher order terms (X squared, X cubed, etc.).
Why does this happen? When all the X values are positive, higher values produce high products and lower values produce low products. So the product variable is highly correlated with the component variable. I will do a very simple example to clarify. (Actually, if they are all on a negative scale, the same thing would happen, but the correlation would be negative).
In a small sample, say you have the following values of a predictor variable X, sorted in ascending order:
2, 4, 4, 5, 6, 7, 7, 8, 8, 8
It is clear to you that the relationship between X and Y is not linear, but curved, so you add a quadratic term, X squared (X2), to the model. The values of X squared are:
4, 16, 16, 25, 49, 49, 64, 64, 64
The correlation between X and X2 is .987–almost perfect.
To remedy this, you simply center X at its mean. The mean of X is 5.9. So to center X, I simply create a new variable XCen=X-5.9.
These are the values of XCen:
-3.90, -1.90, -1.90, -.90, .10, 1.10, 1.10, 2.10, 2.10, 2.10
Now, the values of XCen squared are:
15.21, 3.61, 3.61, .81, .01, 1.21, 1.21, 4.41, 4.41, 4.41
The correlation between XCen and XCen2 is -.54–still not 0, but much more managable. Definitely low enough to not cause severe multicollinearity. This works because the low end of the scale now has large absolute values, so its square becomes large.
The scatterplot between XCen and XCen2 is:
If the values of X had been less skewed, this would be a perfectly balanced parabola, and the correlation would be 0.
Tonight is my free teletraining on Multicollinearity, where we will talk more about it. Register to join me tonight or to get the recording after the call.